The Puzzle Of The Squire's Yeoman
(
CANTERBURY PUZZLES)
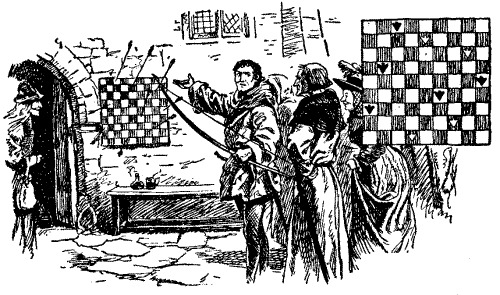
Chaucer says of the Squire's Yeoman, who formed one of his party of pilgrims, "A forester was he truly as I guess," and tells us that "His arrows drooped not with feathers low, And in his hand he bare a mighty bow." When a halt was made one day at a wayside inn, bearing the old sign of the "Chequers," this yeoman consented to give the company an exhibition of his skill. Selecting nine good arrows, he said, "Mark ye, good sirs, how that I shall shoot these nine arrows in such manner that each of them shall lodge in the middle of one of the squares that be upon the sign of the 'Chequers,' and yet of a truth shall no arrow be in line with any other arrow." The diagram will show exactly how he did this, and no two arrows will be found in line, horizontally, vertically, or diagonally. Then the Yeoman said: "Here then is a riddle for ye. Remove three of the arrows each to one of its neighbouring squares, so that the nine shall yet be so placed that none thereof may be in line with another." By a "neighbouring square" is meant one that adjoins, either laterally or diagonally.
Read Answer
Next:
The Nun's Puzzle
Previous:
The Carpenter's Puzzle
 Informational
Informational